Table of Contents
In Chemical Engineering, Convective Mass Transfer Coefficient is a fundamental parameter which quantifies the rate at which mass is transferred between a surface and a fluid. It plays an important role various applications ranging from drying and distillation to absorption and chemical reactions.
Mass Transfer Through Convection – Theory
Mass Transfer through convective involves the transport of mass (usually a species or solute) from one location to another due to the movement of a fluid (liquid or gas). Convective mass transfer is often combined with diffusion, where the species move due to concentration gradients.
Similar to convective heat transfer coefficient, the convective mass transfer are of two types – forced convection mass transfer and free convection mass transfer.
- In free convection mass transfer the motion in the medium is caused by the difference in the density.
- In forced convection mass transfer the motion or flow in the medium is caused by the external source (like a pump, blower or compressor).
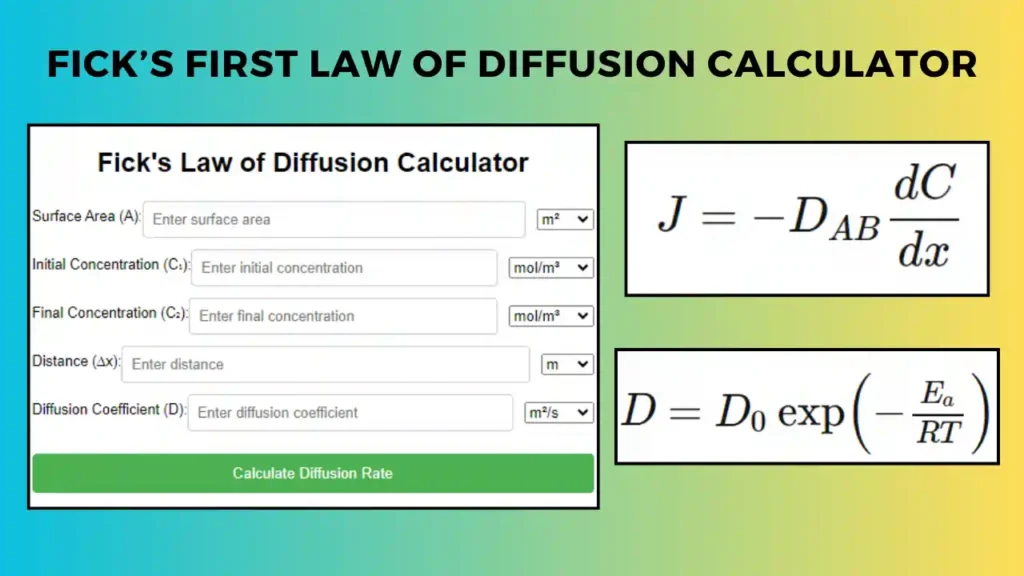
Related: Fick’s First Law of Diffusion Calculator – Molecular Diffusion
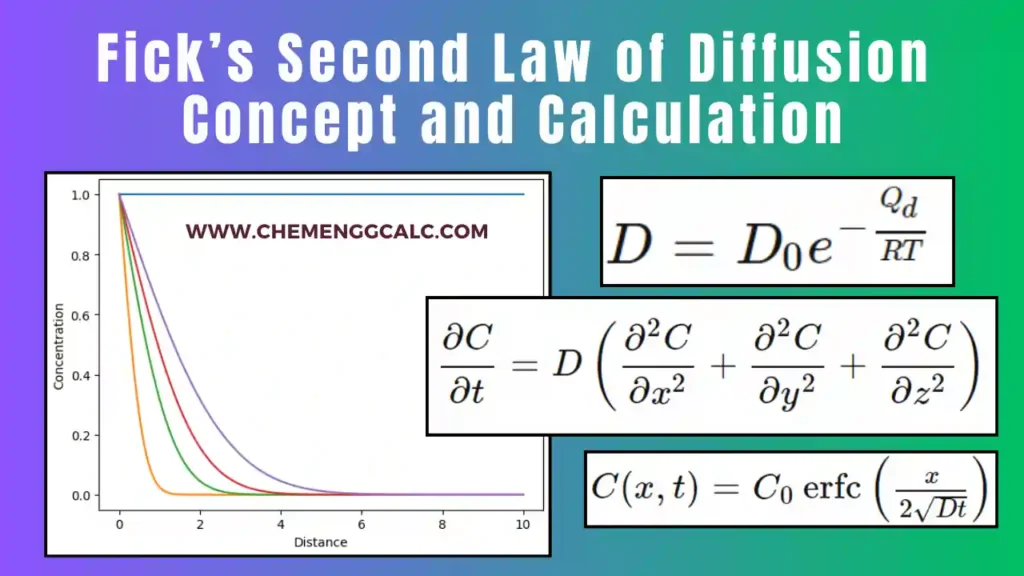
Related: Fick’s Second Law of Diffusion – Concept and Calculation
Convective Mass Transfer Coefficient
The convective mass transfer coefficient, \(k_c\), is a parameter that quantifies the rate at which a species is transferred between a boundary surface (like a solid or liquid surface) and a moving fluid, or between two immiscible fluids.
The convective mass transfer coefficient (\(k_c\)) is given by the equation:
\[N_A = k_c (C_{A,s} – C_{A,\infty})\]
where in S.I units:
- \(N_A\) is the molar flux \(\left(\frac{\text{mol}}{\text{m}^2 \cdot \text{s}}\right)\),
- \(k_c\) is the convective mass transfer coefficient \(\left(\frac{\text{m}}{\text{s}}\right)\),
- \(C_{A,s}\) is the concentration at the phase boundary \(\left(\frac{\text{mol}}{\text{m}^3}\right)\),
- \(C_{A,\infty}\) is the concentration in the bulk fluid \(\left(\frac{\text{mol}}{\text{m}^3}\right)\).
The mass transfer coefficient \(k_c\) depends on the flow conditions (laminar or turbulent), fluid properties, and surface geometry.
Also Read: Thermal Boundary Layer Thickness (δT) for Flat Plate
Also Read: Interactive McCabe-Thiele Diagram Calculator for Binary Distillation
Difference Between Convection and Diffusion Mass Transfer
In mass transfer processes, convection and diffusion are two fundamental mechanisms through which transport of mass takes place, and they operate based on different principles. Here we are showing the key differences between convection and diffusion mass transfer.
| Aspect | Convection | Diffusion |
|---|---|---|
| Mechanism | Bulk movement of fluid driven by external forces (e.g., pumps, fans) or natural effects (e.g., temperature gradients) | Random molecular movement due to concentration gradients |
| Driving Force | Fluid velocity and external forces | Concentration gradient |
| Speed | Generally faster, depending on fluid flow rate | Generally slower, depending on molecular motion |
| Temperature Effect | Significant, as temperature affects fluid density and movement | Less direct impact, though temperature affects molecular speed |
| Rate of Transfer | Higher rate due to fluid movement and mixing | Lower rate, depends on the diffusion coefficient and concentration gradient |
| Typical Applications | Heat exchangers, cooling systems, atmospheric processes | Separation processes, diffusion through membranes, mixing in gases or liquids |
| Dependence on Fluid Properties | Depends on fluid properties like viscosity and density | Depends on molecular size and the medium’s diffusion coefficient |
| Example | In a room with a heater, warm air rises and circulates throughout the room, warming it up. | In a drop of ink placed in still water, the ink slowly spreads out until it’s evenly distributed throughout the water. |
Types of Mass Transfer Coefficients
From the concept of mass-transfer coefficients in terms of flux and concentration gradients, integrating the effects of molecular and turbulent diffusion we get the following equation:
The flux of component A \(( J_{A1}^* )\) through a surface area \( A_1 \) is given by:
\[J_{A1}^* = -\left( D_{AB} + \epsilon_M \right) \frac{d(c_A)}{dz}\]
where:
- \(D_{AB}\) is the molecular diffusion coefficient,
- \(\epsilon_M\) represents the effect of turbulent diffusion or eddy diffusivity,
- \(\frac{d(c_A)}{dz} \) is the concentration gradient.
Integrating this differential equation over the distance \( (z_1 – z_2) \) gives:
\[J_{A1}^* = -\frac{D_{AB} + \epsilon_M}{z_1 – z_2} \left( c_{A1} – c_{A2} \right)\]
here \( c_{A1} \) and \( c_{A2} \) are the concentrations of component A at positions \( z_1 \) and \( z_2 \), respectively.
The mass-transfer coefficient \( k_c’ \) can be defined as:
\[J_{A1}^* = k_c’ \left( c_{A1} – c_{A2} \right)\]
here, \(k_c’ = -\frac{D_{AB} + \epsilon_M}{z_1 – z_2}\)
Here, \( k_c’ \) represents the mass-transfer coefficient, which depends on the diffusion coefficients and the distance over which the transfer occurs.
Hence, flux can be written using a convective mass transfer coefficient.
Flux = (coefficient) (conc. difference)
The mass transfer coefficients can be expressed in different terms as the concentration
1. For Transfer of A through Stagnant B \(( N_B = 0 )\)
For Gases
\[N_A = k_G (p_{A1} – p_{A2})\]
\[N_A= k_y (y_{A1} – y_{A2})\]
\[N_A = k_C (C_{A1} – C_{A2}) \]
where:
- \( k_G \) is the mass transfer coefficient based on partial pressure,
- \( k_y \) is the mass transfer coefficient based on mole fraction,
- \( k_C \) is the mass transfer coefficient based on concentration.
For Liquids
\[N_A = k_x (x_{A1} – x_{A2})\]
\[N_A = k_L (C_{A1} – C_{A2}) \]
where:
- \( k_x \) is the mass transfer coefficient based on mole fraction,
- \( k_L \) is the mass transfer coefficient based on concentration.
2. For Equimolar Counter Transfer \(( N_A = -N_B )\)
For Gases
\[N_A = k_G’ (p_{A1} – p_{A2})\]
\[N_A = k_y’ (y_{A1} – y_{A2}) \]
\[N_A= k_C’ (C_{A1} – C_{A2}) \]
For Liquid
\[N_A = k_x’ (x_{A1} – x_{A2})\]
\[N_A= k_L’ (C_{A1} – C_{A2})\]
where:
- \( k_x’ \) is the mass transfer coefficient based on mole fraction,
- \( k_L’ \) is the mass transfer coefficient based on concentration.
Edition: Revised 2nd Edition, By: R. Byron Bird, Warren E. Stewart, Edwin N. Lightfoot
Comprehensive coverage of transport phenomena, including momentum, heat, and mass transfer. Updated content for better clarity.
Buy on AmazonRelation Between Mass Transfer Coefficients
The relationship between mass transfer coefficients are expressed in terms of the transfer rates across different phases (gas and liquid) and different units (partial pressure, concentration, mole fraction).
Overall Mass Transfer Coefficient
The overall mass-transfer coefficient is the reciprocal of the sum of resistances in both phases. It sums up the effects of mass transfer from individual phases, as seen in gas-liquid systems or in terms of different concentration driving forces.
For Gas-Liquid Mass Transfer
In gas-liquid systems, the overall mass-transfer coefficient \( K_G \) (based on partial pressure) or \( K_L \) (based on liquid concentration) combines the contributions from the gas-phase and liquid-phase resistances.
For Gases:
\[\frac{1}{K_G} = \frac{1}{k_G} + \frac{H}{k_L}\]
where:
- \( K_G \) is the overall mass-transfer coefficient based on gas-phase concentration (or partial pressure),
- \( k_G \) and \( k_L \) are the gas and liquid individual mass-transfer coefficients, respectively.
- \( H \) is the Henry’s law constant, which relates gas concentration to its partial pressure.
For Liquids:
\[\frac{1}{K_L} = \frac{H}{k_G} + \frac{1}{k_L}\]
where:
- \( K_L \) is the overall mass-transfer coefficient based on liquid concentration,
- \( H \) is the Henry’s law constant,
- \( k_G \) and \( k_L \) are the gas and liquid individual mass-transfer coefficients, respectively.
Relationship Between Different Forms of Mass-Transfer Coefficients
The mass-transfer coefficient are also be expressed in different forms as partial pressure, concentration, or mole fraction.
For gas phase mass transfer:
\[k_C = k_G \cdot RT\]
where:
- \( k_C \) is the mass-transfer coefficient based on concentration (mol/m³·s),
- \( k_G \) is the mass-transfer coefficient based on partial pressure (mol/m²·s·Pa),
- \( R \) is the universal gas constant,
- \( T \) is the absolute temperature.
For mole fraction basis:
\[k_y = k_G \cdot P_{\text{total}}\]
where:
- \( k_y \) is the mass-transfer coefficient based on mole fraction,
- \( P_{\text{total}} \) is the total system pressure.
For liquid phase mass transfer
\[k_x = k_L \cdot C_{\text{total}}\]
where:
- \( k_x \) is the mass-transfer coefficient based on mole fraction in the liquid,
- \( C_{\text{total}} \) is the total molar concentration of the liquid.
Also Read: Arrhenius Activation Energy Calculator for two temperatures
Resources
- “Transport Phenomena” by R. Byron Bird, Warren E. Stewart, and Edwin N. Lightfoot.
- “Introduction to Chemical Engineering Thermodynamics” by J.M. Smith, H.C. Van Ness, and M.M. Abbott.
- “Mass Transfer Operations” by Robert E. Treybal.
Disclaimer: The Solver provided here is for educational purposes. While efforts ensure accuracy, results may not always reflect real-world scenarios. Verify results with other sources and consult professionals for critical applications. Contact us for any suggestions or corrections.
- Chemically Complex Materials: The Next Era of Sustainable High-Performance Alloys
- How AI and Self-Driving Labs are Transforming Chemical Engineering in 2026
- New Crystalline Material Breaks 30-Year Limit for CO2 Carbon Capture
- Scientists Turn Plastic Waste and Dead Battery Acid Into H2 Fuel Using Sunlight
- Steam Table Calculator based on IAPWS-IF97 Properties – All 5 Regions Included
