Arrhenius Activation Energy Calculator for two temperatures

The Arrhenius equation is a formula that describes how the rate constant \( k \) of a chemical reaction depends on temperature and activation energy.

To calculate the Arrhenius activation energy (\( E_a \)) using the Arrhenius equation for two different temperatures, here is the following formula:

\[E_a = R \times \left( \frac{\ln(k_2/k_1)}{1/T_1 – 1/T_2} \right)\]

where:

• \(R\) is the ideal gas constant 8.314 \(J/(mol*K)\)
• \(k_1\) and \(k_2\) are the rate constants at temperatures \(T_1\) and \(T_2\) respectively
• \(T_1\) and \(T_2\) are the absolute temperatures in Kelvin.

Related: Performance Equation for Ideal Batch Reactor

Related: Arrhenius Equation Calculator

Arrhenius Activation Energy Calculator for two temperatures

This Activation Energy calculator is helpful for users to determine the activation energy \(𝐸_𝑎\) using the Arrhenius equation for two different temperatures. Users input the rate constants \(k_1\) and \(k_2\) and temperatures \(T_1\) and \(T_2\) (Celsius, Kelvin, or Fahrenheit).

Note: To show the units of k, we assumed second order reaction. However calculation are same for other rates of reactions as well.

Related:  Heat Transfer through Conduction Calculator

Related: Performance Equation for Mixed Flow Reactor

Activation Energy Derivation for two temperatures

Here is the derivation of the activation energy using the Arrhenius equation for two different temperatures.

Starting with the Arrhenius equation for two different temperatures \( T_1 \) and \( T_2 \) with their respective rate constants \( k_1 \) and \( k_2 \):

\[k_1 = A e^{-\frac{E_a}{RT_1}}\]

\[k_2 = A e^{-\frac{E_a}{RT_2}}\]

Here, \( A \) is the pre-exponential factor, \( E_a \) is the activation energy, \( R \) is the ideal gas constant, and \( T \) is the absolute temperature.

Now, taking the natural logarithm of both equations:

\[\ln(k_1) = \ln(A) – \frac{E_a}{RT_1}\]

\[\ln(k_2) = \ln(A) – \frac{E_a}{RT_2}\]

Subtracting the second equation from the first:

\[\ln(k_1) – \ln(k_2) = \left( \frac{E_a}{RT_2} \right) – \left( \frac{E_a}{RT_1} \right)\]

Simplify the equation using the properties of logarithms, we get:

\[\ln\left(\frac{k_1}{k_2}\right) = – \frac{E_a}{R} \left( \frac{1}{T_1} – \frac{1}{T_2} \right)\]

Now, solving for \(E_a\)

\[E_a = -R \times \left( \frac{\ln\left(\frac{k_1}{k_2}\right)}{\frac{1}{T_1} – \frac{1}{T_2}} \right)\]

Note that the negative sign will cancel out because ( \(T_2\) > \(T_1\) ) implies \(( \frac{1}{T_1} – \frac{1}{T_2} < 0 )\) and \(( \ln(k_1) – \ln(k_2) )\) will also be negative if \(( k_1 < k_2 )\).

Related: PFR and CSTR in Series or Parallel Combination for a single reaction

Chemical Reaction Engineering

Edition: 3rd Edition, By: Octave Levenspiel

A classic textbook covering the principles of chemical reaction engineering with detailed analysis of reactor design and kinetics.

Buy on Amazon

Example Problem on Activation Energy

At a temperature of \(600 \, \text{K}\), the rate constant of a chemical reaction is \((2.75 \times 10^{-8} \) \(\text{M}^{-1}\text{s}^{-1})\). When the temperature is increased to \(800 \, \text{K}\), the rate constant for the same reaction is \((1.95 \times 10^{-7} \, \text{M}^{-1}\text{s}^{-1})\). What is the activation energy of this reaction?

Given,

• \( T_1 = 600 \, \text{K} \)
• \( k_1 = 2.75 \times 10^{-8} \, \text{M}^{-1}\text{s}^{-1} \)
• \( T_2 = 800 \, \text{K} \)
• \( k_2 = 1.95 \times 10^{-7} \, \text{M}^{-1}\text{s}^{-1} \)

using Arrhenius Activation Energy equation for two temperatures:

\( \ln\left(\frac{k_1}{k_2}\right) = \left(-\frac{E_a}{R}\right) \left(\frac{1}{T_1} – \frac{1}{T_2}\right) \)

Substituting the given values in the equation for the value of \( E_a \)

\( \ln\left(\frac{2.75 \times 10^{-8}}{1.95 \times 10^{-7}}\right) = \left(-\frac{E_a}{8.314}\right) \left(0.00041 \right) \)

\( \ln(0.141) = E_a \cdot (-0.0000493) \)

\( E_a = \frac{-1.958}{-0.0000493} \, \text{J} \cdot \text{mol}^{-1} \)

\( E_a = 39085 \, \text{J} \cdot \text{mol}^{-1} \)

The activation energy of the reaction is approximately \( 39085 \, \text{J} \cdot \text{mol}^{-1} \).

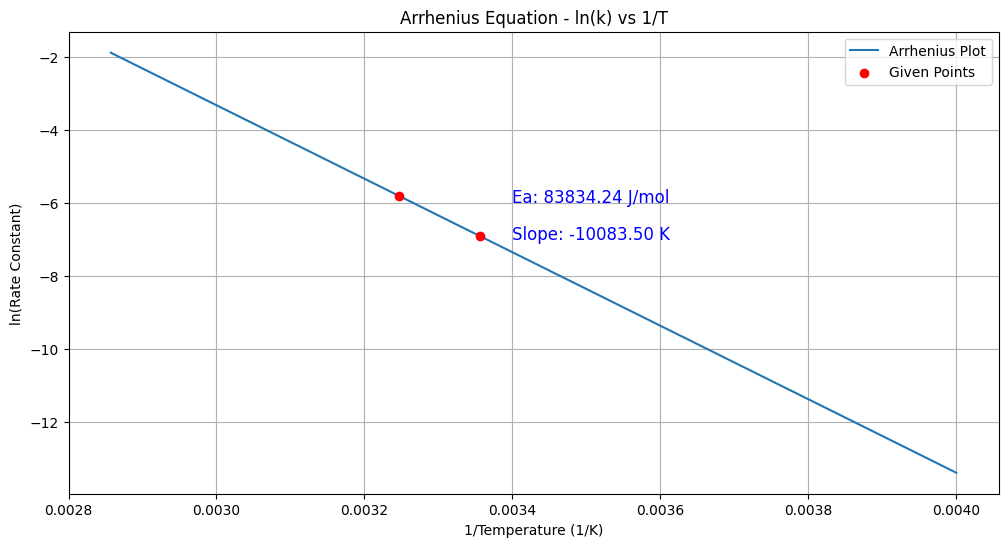
Python Code for Arrhenius Equation – Activation Energy

This python code calculates the activation energy for a chemical reaction using the Arrhenius equation for two different temperatures. The slope of plot is related to the activation energy.

Note: This Python code solves the specified problem for 1st order reaction. Users can copy the code and run it in a suitable Python environment. By adjusting the input parameters, and observe how the output changes accordingly.

import numpy as np
import matplotlib.pyplot as plt

# Constants
R = 8.314  # Gas constant in J/(mol·K)

# Function to calculate activation energy
def calculate_activation_energy(T1, T2, k1, k2):
    ln_k_ratio = np.log(k2 / k1)
    T1_inv = 1 / T1
    T2_inv = 1 / T2
    Ea = -ln_k_ratio * R / (T2_inv - T1_inv)
    return Ea

# Example temperatures (in Kelvin) and rate constants
T1 = 298  # Temperature 1 in Kelvin
T2 = 308  # Temperature 2 in Kelvin
k1 = 1e-3  # Rate constant at T1
k2 = 3e-3  # Rate constant at T2

# Calculate activation energy
Ea = calculate_activation_energy(T1, T2, k1, k2)
slope = -Ea / R
print(f'Activation Energy: {Ea:.2f} J/mol')
print(f'Slope: {slope:.2f} K')

# Generate data for plotting
temperatures = np.linspace(250, 350, 100)
inverse_temperatures = 1 / temperatures
ln_rate_constants = lambda T: np.log(k1 * np.exp(-Ea / R * (1 / T - 1 / T1)))

# Calculate ln(k) for the range of temperatures
ln_rate_constants_values = ln_rate_constants(temperatures)

# Plotting ln(k) vs 1/T
plt.figure(figsize=(12, 6))
plt.plot(inverse_temperatures, ln_rate_constants_values, label='Arrhenius Plot')
plt.scatter([1/T1, 1/T2], [np.log(k1), np.log(k2)], color='red', zorder=5, label='Given Points')

# Adding annotations for slope and activation energy
plt.text(0.0034, -7, f'Slope: {slope:.2f} K', fontsize=12, color='blue')
plt.text(0.0034, -6, f'Ea: {Ea:.2f} J/mol', fontsize=12, color='blue')

# Labels and title
plt.xlabel('1/Temperature (1/K)')
plt.ylabel('ln(Rate Constant)')
plt.title('Arrhenius Equation - ln(k) vs 1/T')
plt.legend()
plt.grid(True)
plt.show()

Output:

Resources:

• Chemical Reactor Analysis and Design Fundamentals by Rawlings and Ekerdt
• Elements of Chemical Reaction Engineering by Fogler
• “Chemical Reaction Engineering“ by Octave Levenspiel

Disclaimer: The Solver provided here is for educational purposes. While efforts ensure accuracy, results may not always reflect real-world scenarios. Verify results with other sources and consult professionals for critical applications. Contact us for any suggestions or corrections.